Why does water stop flowing from a closed jug so quickly?

Have you ever tried to fill your water bottle from a water jug with a spout, leaving the jug lid sealed?

If so, you probably noticed the flow rate quickly falls to zero. Once the lid is loosened, the flow rate returns to normal and your water bottle fills after a short time. This mundane situation illustrates some interesting physics, and unexpected math.

Physics concepts: fluid mechanics, Poiseuille's Law, Ideal Gas Law.

Mathematical tools: calculus, differential equations, separation of variables.

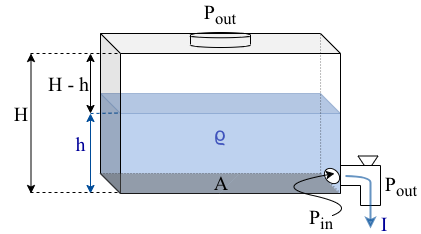
The situation is depicted in Fig. 1 to the right.

We know from experience just how significant the lid is when filling a water bottle. If it's loose enough to allow free airflow, everything's great. At least, until the water height, h, gets too low. But if you leave the lid tight, you'll be lucky to get any more than a few sips out.

The main physical reason is pressure.

When the lid is open, pressure on the water's surface is atmospheric, matching the 'back-pressure' applied at the spout. So, the pressure difference between the inside and outside of the spout is purely due to the water's weight. That's why the water flows quite well, but then slows as the water height decreases. There is another consideration, because the spout introduces some resistance to the flow -- we won't worry about it too much here since the resistance is a constant, unaffected by the lid being open or closed.

With the lid closed, the pressure difference is quite different. There is still an atmospheric 'back-pressure' at the outside of the spout. However, now the water's surface is not exposed to the atmosphere. Even if the lid was closed recently, so that the air pressure inside the jug begins at atmospheric pressure, the air pressure will quickly decrease as the water's surface forces it to expand. (I think we can assume isothermal -- constant temperature -- expansion in this case.) The water will fall only to the point at which the pressure of the water's weight and air trapped in the jug matches the external atmospheric pressure.

So, how far is that exactly? And how long does it take for the water to fall that much, compared to the open lid case?

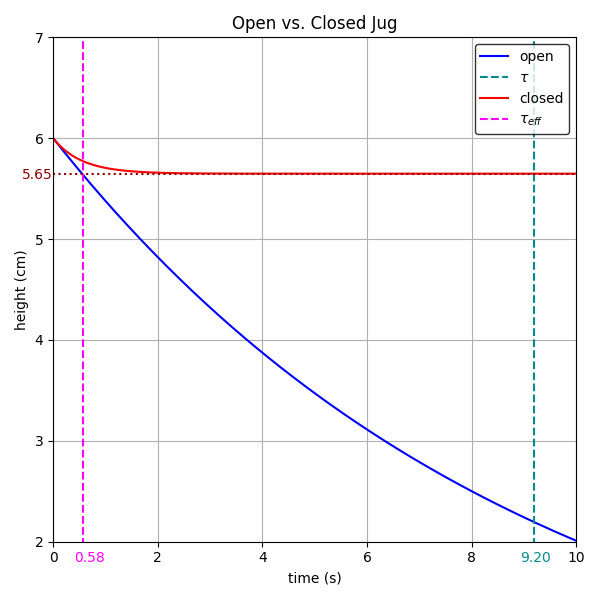
Those answers depend on specifics, like your jug geometry and local atmospheric pressure. But, making some reasonable assumptions, the math reveals some typical answers: the water level may fall roughly 0.1 cm, and it could take a very short time like 0.1 sec. On the other hand, the open lid case allows the water to empty all the way down to the spout intake. If you need a height of 1 cm of water from the jug, the closed case only gets you about 10% of the way there even if you wait for a long time, while the open case will give you that much water in less than 2 seconds.

The main point is illustrated in Fig. 2 below. It shows the height of the water, as varying with time, for each case. The minimum height for the closed lid case is shown also, as well as the 'characteristic time' for each case. One catch with this plot: I used a low atmospheric pressure, just 0.1 atm, because otherwise the closed lid case is on too short of a timescale to really compare with the open lid case. (The open lid case is unaffected by the value of the atmospheric pressure.)

Want all the details?

Full notes, mathematical details [PDF, 4 pages]

Contact me for Python code [plotting with Numpy and Matplotlib]